There is a lot in the topic of exponential variables, but today we are going to focus x⁰ and how students think about it.
This last week, we gave 400 some students the following question.

And I’m sure many of you could guess their answers. We’ve got the correct answer of 1 at 59%, 0 at 30%, 3 at 6% and the remaining 5% were a variety of one off attempts.
But hold on, some of the answers have a little bit more information from the students and how they might be thinking about the problem. We’ve got things like “undefined”,”nothing or just 0″, “1/0” and “0/3”. And its really compelling to see so many students write 0. For context, the same group of students got 1/9 for 3⁻² 80% of the time.
We had a theory. Myself, Patrick Callahan and Phil Daro have been throwing around this idea of “invisible ones” for the past couple of months. And the idea is that in our math syntax, there are a bunch of 1s we don’t write out and instead leave a blank space for. Since we are currently talking about exponential variables, I’ll give an exponential example. We write things like 3 x 3, instead of 3¹ x 3¹. So, students know that 3 x 3 = 9, but have to then later learn that 3¹ x 3¹ = 3². Often confusing whether you are supposed to add, subtract, multiply or divide the exponential term.
And when thinking about 3⁰, I was thinking this may be an invisible one problem. Luckily you don’t have to believe me, because we were able to test it out.
Students are generally taught that an exponent is how many times to multiply the number by. For example, 5³ is 5 x 5 x 5. You multiply 5 times itself 3 times. And then for negative exponents, you just do 1 over (5 x 5 x 5). But what happens if you multiply five by itself 0 times?
“undefined”, “nothing”, “just 0” may come to mind! It makes sense, we aren’t doing anything. And that is where I think the invisible 1 comes in. What if 5³ isn’t 5 x 5 x 5, but 1 x 5 x 5 x 5. And 5³ isn’t multiplying 5 by itself three times, but rather multiplying 1 by 5 three times.
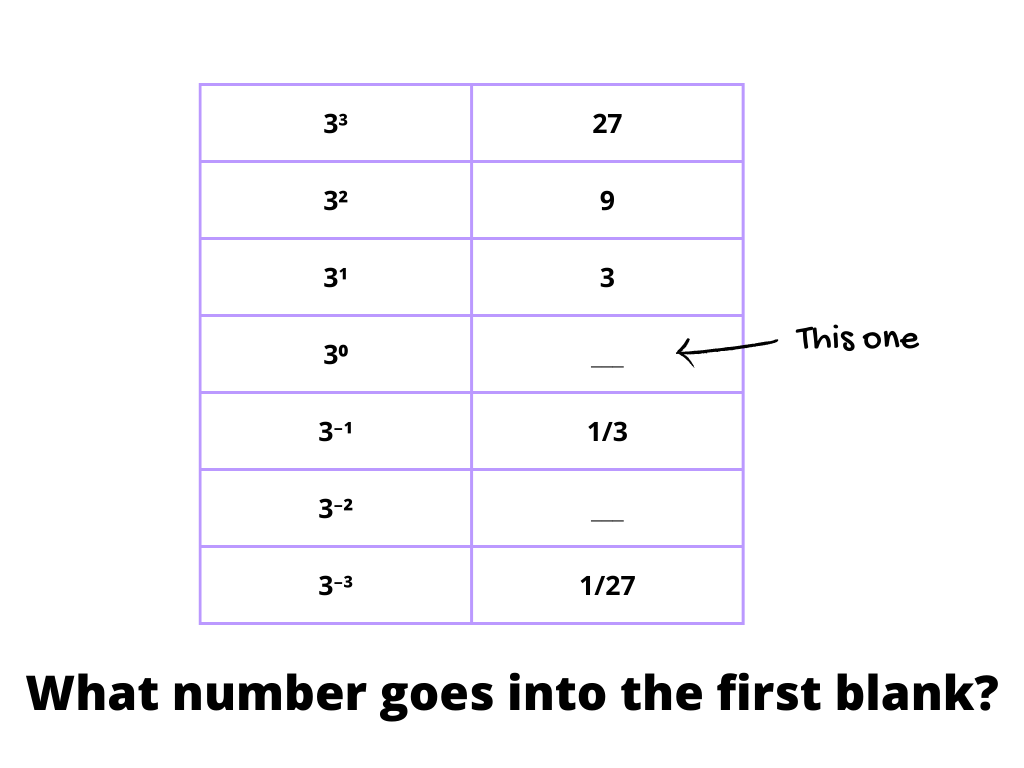
With that in mind, we made a re-engagement exercise that looked at the pattern of exponents.
| Exponential Form | Expanded Pattern | Pattern W/O Invisible 1 | Result |
| 3³ | 1 x 3 x 3 x 3 | 3 x 3 x 3 | 27 |
| 3² | 1 x 3 x 3 | 3 x 3 | 9 |
| 3¹ | 1 x 3 | 3 | 3 |
| 3⁰ | 1 | 1 |
And then students discussed the linear pattern in the table in the “Expanded Pattern” Column. “Every time you increase the exponent by 1, you multiply by 3 again.” However, here we see that when you get to zero, you aren’t left with a blank space in the pattern, but 1.
And then we got lucky. Some of the teachers we are working taught the normal table pattern where you just talk about the multiplicative pattern of multiplying or dividing by the number to go up and down the tables beforehand and some used our re-engagement exercises. (You can too: Teacher Facing and Student Facing)
And here were the results after giving the same problem, but using a table of 4s instead of 3s.
| No Re-Engagement Exercise | Re-Engagement Exercise Used | |
| 3⁰ = 1, 4⁰ = 1 | 79.71% | 95% |
| 3⁰ = 1, 4⁰ = 0 | 20.29% | 5% |
| 3⁰ = 0. 4⁰ = 1 | 38.46% | 67.44% |
| 3⁰ = 0, 4⁰ = 0 | 61.76% | 32.56% |
We saw both a massive jump in the students who were getting 0, now getting 1. And better retention in the students who got 1 the first time.
And here are the same results in visual form with the right answer as green, answering 0 as blue, and the addition of answers outside of 0 or 1 as yellow..

Overall, it was a big win with all classrooms showing improvement. And the start of some evidence that the idea behind showing students that exponents are actually multiplying by 1 that many times, rather than “themselves”.