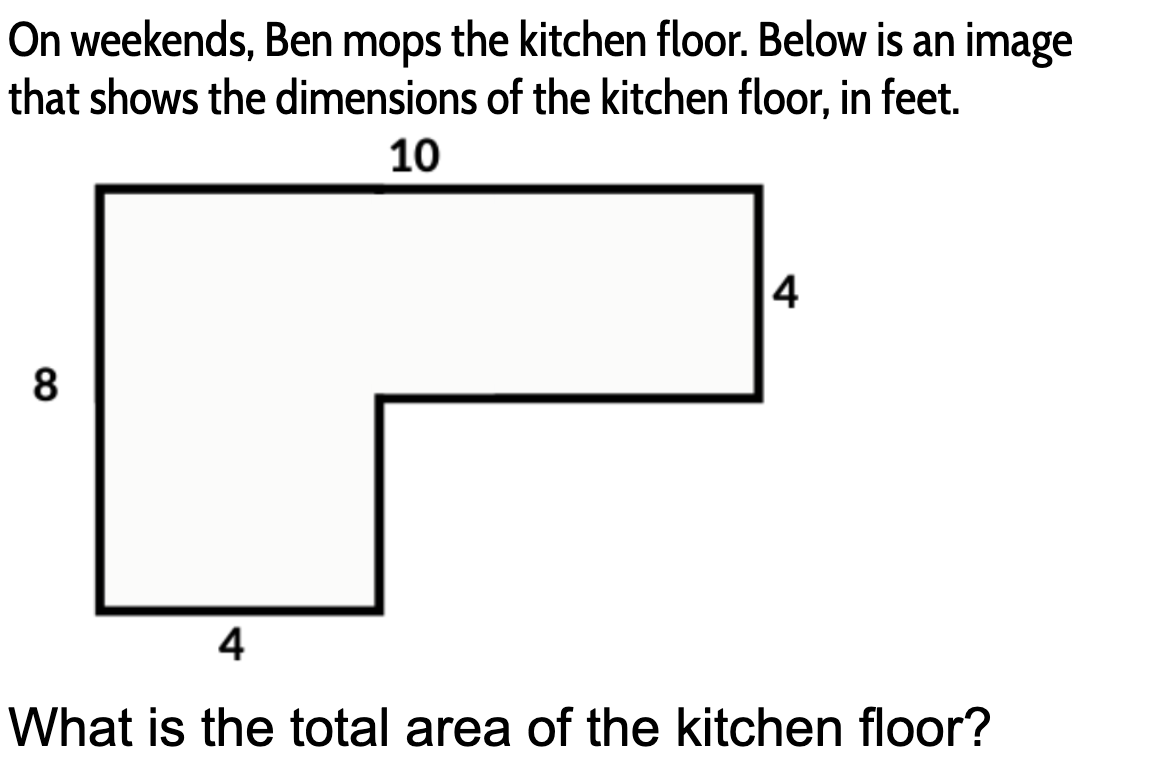
First off, I want to thank Annie Fetter for challenging me and a bunch of other people to dig deeper almost a year ago. In an attempt to do the same for you as Annie did for me, I’m going to ask you to solve a simple math question for me.

After you solve it, read on.
So, the solving piece of this doesn’t really matter because that isn’t what this post is about. Instead, I want you to go back and think about what your first step was to solve the problem.
If you are like me, you answer: “I multiplied 4 and 10” or “I calculated the missing sides. 10 – 4 = 6!”. And here is where Annie challenged all of us. She called us out, that was not our first step. We likely read the problem, picked out that we needed to solve for area, thought to ourselves that area is length times width, and potentially a host of other things.
The first step we all do is to make sense of the problem. And Annie talks a lot about that using “Notice and Wonder”. And while doing some work for a school, we found that a lot of the students weren’t making sense of the questions they were given. And that got me thinking about how we make sense of questions.
And so, I wanted to break things down into types of sense making in order to make the idea of making sense of a question more concrete and actionable for students and their educators.
Making Sense of Units
In this case, let’s start with a much more difficult geometry problem. What if we wanted to calculate the volume of a shape similar to the Washington Monument.

To solve this, you’ll need to know how to decompose this shape, calculate the volume of a rectangular prism and of a pyramid and then add those volumes back together.
But, if we just consider units, we can actually much more easily make sense of this item if we just make sense of the units. We need cubic feet and we are given feet. So, we know we are going to multiply some amount of feet with more feet and then some more feet again! We’ve suddenly limited the possibilities down substantially. That takes away adding the feet together or multiplying them all together (that would be quartic feet). And all we’ve done so far is make sense of the units. That’s pretty incredible! Even better, making sense of units is a strategy that will always work on any math and science problem. Not all the answers are correct, but not because of the units rather because they don’t make sense contextually!
Making Sense of Numbers Contextually
Using the same problem above, what if I gave you a few answers to some questions.
144 cubic feet, 192 cubic feet, 168 cubic feet, 16 cubic feet, and 160 cubic feet.
These are all volumes, but of what?
This is contextual sense making of numbers. What are these numbers? What do they represent in the problem? This holds true for things like the given quantities: 4 ft for example being the length of the bottom of the rectangular prism. Or derived quantities, 4 ft x 4 ft x 9 ft = 144 cubic feet, which is the volume of the rectangular prism.
So, now we can add making sense of numbers in context to the layer of making sense of units. To see that 192 cubic feet is the volume of a rectangular prism with the same height as this shape. (4 x 4 x (9 + 3) = 192 cubic feet). 16 cubic feet is the volume of the pyramid, and 160 cubic feet is the volume of the rectangular pyramid plus the volume of the pyramid or just the volume of the shape we can see. I’ll leave it to you to figure out what 168 cubic feet is. (if you need a hint: pretend you don’t have a way to get the volume of the pyramid, how might you estimate the volume of this shape?)
Making Sense of Mathematical Language
This one is a very mathematically focused. In the first question I showed you, it asked for the area. What is area? In this context, its a math term that defines the size of a surface. Or more formally:
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or plane area refers to the area of a shape or planar lamina, while surface area refers to the area of an open surface or the boundary of a three-dimensional object.
In any case, to interpret the problem correctly, you need to comprehend what area refers to. We often see students solve the correct perimeter (another mathematical term) instead of the area. They correctly calculate the missing sides and then calculate the perimeter instead.
In that case, if you didn’t know what the word referred to, units would not have helped you. So, in this case, we are looking at making sense through comprehension of mathematical terms. We also see this come up in understanding what variables refer to what in a mathematical formula.
Making Sense of Variables
A = LxW, a^2 + b^2 = c^2, SOHCAHTOA, y = mx+b, e^i*pi + 1 = 0, etc.
There are a variety of mathematical laws that we teach our students from the formula for area of a square to the Pythagorean theorem to the quadratic formula. All these formulas are full of variables, but its up to us to make sense of those variables before we use them.
For example, when do you use the Pythagorean theorem and what does a,b and c stand for. Often times, we see students use a^2 + b^2 = c^2 in our assessments, but with non-right triangles or the a and b are the two sides we have. Being able to make sense of what these variables mean and how to use them is key to being able to use these properties. Are you and your students making sense of the variables?
In Conclusion
These are just some ideas for a start of the different kinds of sense making out there. I think there are likely many more of them. Making Sense of Mathematical Structures, Patterns, Space (as in 1d,2d,3d), Images/Graphs/Tables, reasonableness etc. In addition, I think there are Math Language Routines that could be utilized to help in each specific realm of sense making and instead of having to take on sense making as a whole, perhaps we can take it one step at a time.
Let me know if you think of any other types of sense making! Reach out to me on Twitter or LinkedIn. Or feel free to contact us here.